My normal routine for this time of year is to prepare new What Do You Notice? posters for my upcoming Family Math Night events. So that’s what I’m doing. But this time, I’m preparing the posters for a remote learning environment.

These low floor, high ceiling activities actually work well in this new landscape. Students can share out thoughts and ideas during a group Math Talk. And, of course, they can still be a great way to engage families through a Family Math Night at Home activity. Pose the What Do You Notice? poster at the beginning of the week to give families an opportunity to work on it together then have students share-out at the end of the week.

Here’s my latest poster:

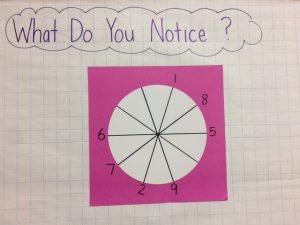
Title: Number Wheel

Skills
K-2: number recognition, counting, geometry
3-5: addition/subtraction, patterns, odd numbers

Background Information
This is a fun puzzle where the main objective is to figure out where the three missing numbers of the “spokes” are placed. Since the numbers 1, 2, 5, 6, 7, 8, and 9 are visible, the missing numbers would be 3, 4, and 10 (assuming we are using the consecutive numbers 1 – 10. There is an alternate solution for the missing numbers 0, 3, and 4 which is described below).

Younger students may notice a circle, a square, and (10) “triangular” pie pieces. They will probably notice that some of the spokes are missing numbers. Starting at 1 and counting on, they may discover the three missing numbers: 3, 4, and 10. It would be interesting to ask them where they think the numbers go on the wheel and why they chose those specific spokes.

Older students will quickly notice three missing numbers. With a little bit of guidance, they should be able to notice some patterns that can help them determine where the missing numbers should be placed.

One possibility: Any two adjacent numbers add up to the same amount as the two adjacent numbers on the opposite spokes. For example, 1 + 8 = 7 + 2. Or 8 + 5 = 6 + 7. Therefore, the numbers on the spokes would read: 1, 8, 5, 4, 9, 2, 7, 6, 3, and 10.

Another possibility: The difference between the adjacent numbers is always an odd number. For example, the difference between 1 and 8 is 7. The difference between 8 and 5 is 3 and so on. Keeping with this pattern, the placement of the numbers would be the same as the first possibility above but could also be: 1, 8, 5, 10, 9, 2, 7, 6, 3, and 4.

Something to think about: If a student decides that all the missing numbers need to be single-digit numbers, then the numbers missing would be 0, 3, and 4. Students could plug these numbers in to the second possibility listed above to get 1, 8, 5, 0, 9, 2, 7, 6, 3, 4 or 1, 8, 5, 4, 9, 2, 7, 6, 3, 0. The difference between each number is still an odd number.

Making the wheel: I used an 8″ diameter circle then used a protractor to divide the circle into ten 36˚ sections.

As many of you know, I have a lot of other What Do You Notice? posters on our website. Each one would make a great addition to your remote learning lesson plans.